2010年 AIME II 数学邀请赛真题
问题 1
设为所有数字都是偶数且没有两个数字相同的数![]() 的最大整数倍。求除以时的余数。
的最大整数倍。求除以时的余数。 ![]()
![]()
![]()
问题 2
![]() 在单位正方形 内部随机选取一点
在单位正方形 内部随机选取一点![]() 。设表示从到 的最近边的
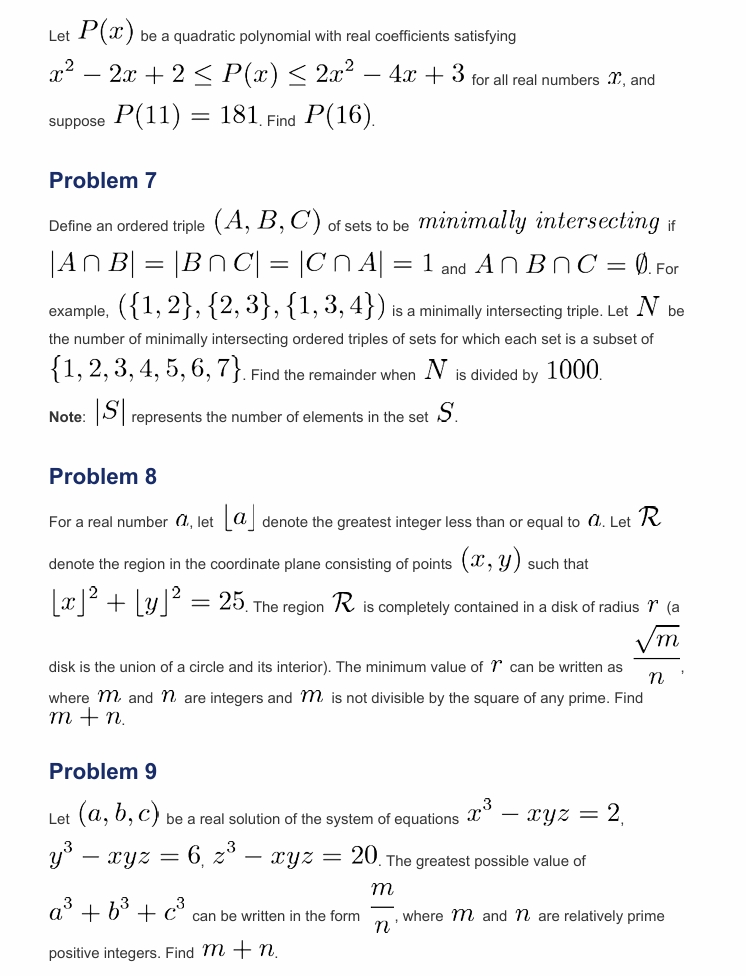
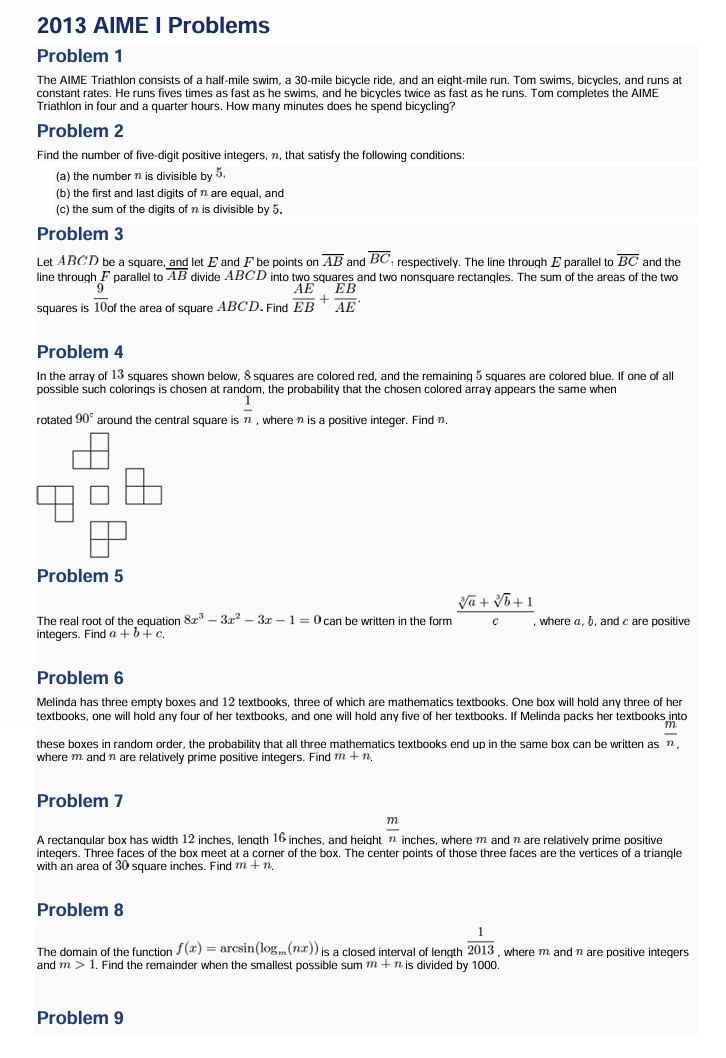
。设表示从到 的最近边的![]() 距离。等于 的概率,其中和为互质正整数。求。
距离。等于 的概率,其中和为互质正整数。求。 ![]()
![]()
![]()
![]()
![]()
![]()
![]()
问题 3
设是所有因数(不一定不同)![]() 的乘积,其中和是满足的整数。找出能够整除的最大正整数。
的乘积,其中和是满足的整数。找出能够整除的最大正整数。 ![]()
![]()
![]()
![]()
![]()
![]()
![]()
问题4
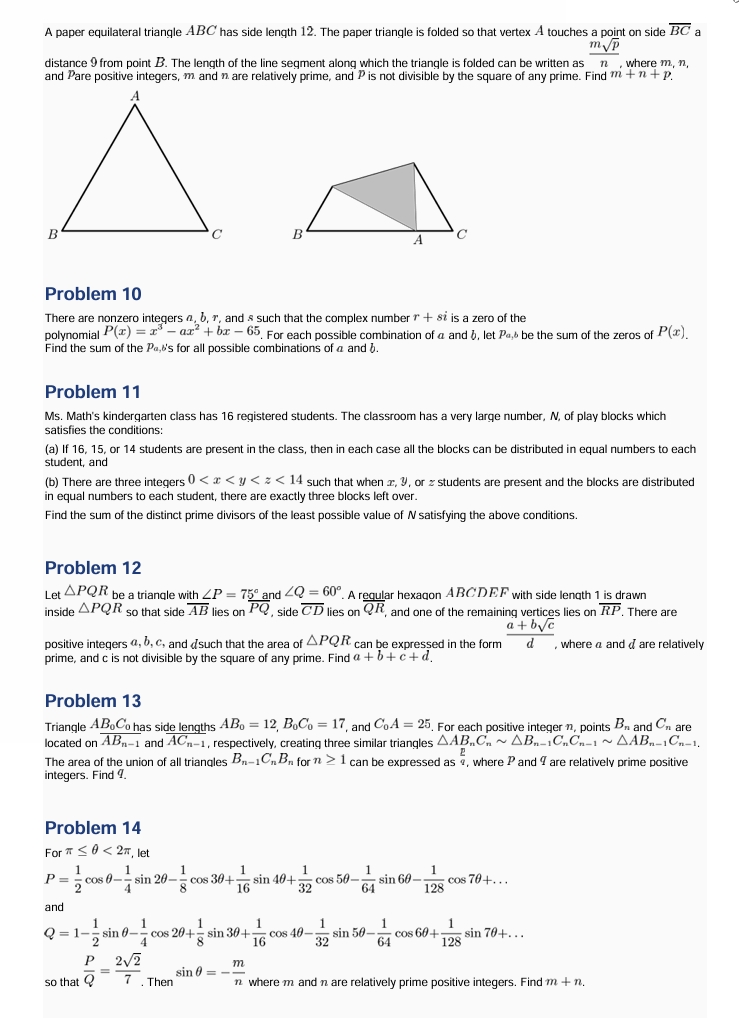
戴夫到达一个机场,该机场有 12 个登机口,排列成一条直线,![]() 相邻登机口之间的距离恰好为英尺。他的登机口是随机分配的。在登机口等待后,戴夫被告知登机口已更改为另一个登机口,同样是随机的。假设戴夫步行
相邻登机口之间的距离恰好为英尺。他的登机口是随机分配的。在登机口等待后,戴夫被告知登机口已更改为另一个登机口,同样是随机的。假设戴夫步行![]() 几英尺或更短时间到达新登机口的概率为分数
几英尺或更短时间到达新登机口的概率为分数![]() ,其中
,其中![]() 和
和![]() 是互质正整数。求
是互质正整数。求![]() 。
。
问题5
正数![]() 、
、![]() 、 和
、 和![]() 满足
满足![]() 和
和![]() 。求
。求![]() 。
。
问题 6
寻找最小的正整数![]() ,其特性是多项式
,其特性是多项式![]() 可以写成两个具有整数系数的非常数多项式的乘积。
可以写成两个具有整数系数的非常数多项式的乘积。
问题 7
设![]() ,其中
,其中![]() ,
,![]() 和
和![]() 为实数。存在一个复数,
为实数。存在一个复数,![]() 使得 的三个根分别
使得 的三个根分别![]() 为
为![]() ,
,![]() 和
和![]() ,其中
,其中![]() 。求
。求![]() 。
。
问题 8
设![]() 是非空集的有序对的数量
是非空集的有序对的数量![]() ,并
,并![]() 具有以下属性:
具有以下属性:
 ,
, ,
,- 元素的数量
 不是 的元素
不是 的元素 ,
, - 元素的数量
 不是 的元素
不是 的元素 。
。
寻找![]() 。
。
问题 9
设![]() 为正六边形。设
为正六边形。设![]() 、
、![]() 、
、![]() 、
、![]() 、
、![]() 和分别为边、、、、和
和分别为边、、、、和![]() 的中点。线段、、、、 和构成一个较小的正六边形。设小六边形的面积与 的面积之比用分数表示,其中和是互质正整数。求。
的中点。线段、、、、 和构成一个较小的正六边形。设小六边形的面积与 的面积之比用分数表示,其中和是互质正整数。求。 ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
问题 10
求出具有整数系数和整数零点的二次多项式的数量![]() ,使得
,使得![]() 。
。
问题11
将T 网格定义为![]() 满足以下两个属性的矩阵:
满足以下两个属性的矩阵:
- 其中恰好有五个条目是
 ,其余四个条目是
,其余四个条目是 。
。 - 在八行、八列、八条长对角线(长对角线为
 和
和 )中,最多只有一条线的三个元素相等。
)中,最多只有一条线的三个元素相等。
找出不同T 型网格的数量。
问题 12
两个不全等的整数边等腰三角形周长和面积相等。两个三角形底边的长度比为![]() 。求出它们公共周长的最小可能值。
。求出它们公共周长的最小可能值。
以下是我们为您整理的全英版pdf真题:


扫码免费获取完整版真题+解析~~~
我们整理了近十年全部AMC备赛资料,包括AMC8/10/12/AIME的历年真题和答案详解,备赛书籍、知识点地图、备赛公式等,
免费提供给备赛的考试使用,扫码下载即可⇓




 表示为
表示为

![\[m^{x_0} = \sum_{k = 1}^{2011} m^{x_k}.\]](https://latex.artofproblemsolving.com/3/7/c/37c3a4d8143a7bc88f260f55b43ac3f3fbb7678e.png)
![[asy] unitize(1 cm); 成对翻译; 对[] A,B,C,U,V,W,X,Y,Z; A[0] = (1.5,2.8); B[0] = (3.2,0); C[0] = (0,0); U[0] = (0.69*A[0] + 0.31*B[0]); V[0] = (0.69*A[0] + 0.31*C[0]); W[0] = (0.69*C[0] + 0.31*A[0]); X[0] = (0.69*C[0] + 0.31*B[0]); Y[0] = (0.69*B[0] + 0.31*C[0]); Z[0] = (0.69*B[0] + 0.31*A[0]); 翻译 = (7,0); A[1] = (1.3,1.1) + 平移; B[1] = (2.4,-0.7) + 平移; C[1] = (0.6,-0.7) + 平移; U[1] = U[0] + 平移; V[1] = V[0] + 平移; W[1] = W[0] + 平移; X[1] = X[0] + 平移; Y[1] = Y[0] + 平移; Z[1] = Z[0] + 平移; 绘制(A[0]--B[0]--C[0]--循环);绘制(U[0]--V[0],虚线);绘制(W[0]--X[0],虚线);绘制(Y[0]--Z[0],虚线);绘制(U[1]--V[1]--W[1]--X[1]--Y[1]--Z[1]--循环);绘制(U[1]--A[1]--V[1],虚线);绘制(W[1]--C[1]--X[1]);绘制(Y[1]--B[1]--Z[1]);点(“$A$”,A[0],N);点(“$B$”,B[0],SE);点(“$C$”,C[0],SW);点(“$U$”,U[0],NE);点(“$V$”,V[0],NW);点(“$W$”,W[0],NW);点(“$X$”,X[0],S);点(“$Y$”,Y[0],S);点(“$Z$”,Z[0],NE);点(A[1]);点(B[1]);点(C[1]);点(“$U$”,U[1],NE);点("$V$",V[1],NW);点("$W$",W[1],NW);点("$X$",X[1],dir(-70));点("$Y$",Y[1],dir(250));点("$Z$",Z[1],NE);[/asy]](https://latex.artofproblemsolving.com/4/4/f/44fd6e9fb5acf1d28fe1e2acd699bd5be1230685.png)

![[asy] 对 S1 = (20, 20), S2 = (-20, 20), S3 = (-20, -20), S4 = (20, -20); 对 M1 = (S1+S2)/2, M2 = (S2+S3)/2, M3=(S3+S4)/2, M4=(S4+S1)/2; 对 Sp1 = (7.5, 7.5), Sp2=(-7.5, 7.5), Sp3 = (-7.5, -7.5), Sp4 = (7.5, -7.5); 绘制(S1--S2--S3--S4--循环); 绘制(Sp1--Sp2--Sp3--Sp4--循环); 绘制(Sp1--M1--Sp2--M2--Sp3--M3--Sp4--M4--循环); [/asy]](https://latex.artofproblemsolving.com/2/8/6/2862b9fac9f2c88c10b30e3908cf4ac1d5f62115.png)


![[asy] 导入cse5;单位尺寸(6mm);默认笔(线宽(.8pt));点因子 = 8;路径笔=黑色;对 A = (0,0);对 B = 2*dir(54),C = 2*dir(126),D = 2*dir(198),E = 2*dir(270),F = 2*dir(342);对 G = 3.6*dir(18),H = 3.6*dir(90),I = 3.6*dir(162),J = 3.6*dir(234),K = 3.6*dir(306);对 M = 6.4*dir(54),N = 6.4*dir(126),O = 6.4*dir(198),P = 6.4*dir(270),L = 6.4*dir(342);对[]点状 = {A,B,C,D,E,F,G,H,I,J,K,L,M,N,O,P};D(A--B--H-- M); D(A--C--H--N); D(A--F--G--L); D(A--E--K--P); D(A-- D--J--O); D(B--G--M); D(F--K--L); D(E--J--P); D(O--I-- D); D(C--I--N); D(L--M--N--O--P--L); 点(虚线);[/asy]](https://latex.artofproblemsolving.com/3/3/a/33abde80848746d240bfc392b8997b80cf3bccd5.png)
![[asy]import cse5; unitize(10mm); pathpen=black; dotfactor=3; pair A = (0,0), B = (3.8,0), C = (5.876,1.564), D = (2.076,1.564), E = (0,3.8), F = (3.8,3.8), G = (5.876,5.364), H = (2.076,5.364), M = (1.9,0), N = (5.876,3.465); pair[] dotted = {A,B,C,D,E,F,G,H,M,N}; D(A--B--C--G--H--E--A); D(E--F--B); D(F--G); pathpen=dashed; D(A--D--H); D(D--C); dot(dotted);标签(“$A$”,A,SW);标签(“$B$”,B,S);标签(“$C$”,C,SE);标签(“$D$”,D,NW);标签(“$E$”,E,W);标签(“$F$”,F,SE);标签(“$G$”,G,NE);标签(“$H$”,H,NW);标签(“$M$”,M,S);标签(“$N$”,N,NE); [/asy]](https://latex.artofproblemsolving.com/a/f/1/af154db9d25256bd90239e9f4e37253b234659e0.png)


 有正整数
有正整数

![[asy] 绘制((0,0)--(1,0)--(1,1)--(0,1)--(0,0)); 绘制((2,0)--(2,2)--(3,2)--(3,0)--(3,1)--(2,1)--(4,1)--(4,0)--(2,0)); 绘制((1,2)--(1,4)--(0,4)--(0,2)--(0,3)--(1,3)--(-1,3)--(-1,2)--(1,2)); 绘制((-1,1)--(-3,1)--(-3,0)--(-1,0)--(-2,0)--(-2,1)--(-2,-1)--(-1,-1)--(-1,1));绘制((0,-1)--(0,-3)--(1,-3)--(1,-1)--(1,-2)--(0,-2)--(2,-2)--(2,-1)--(0,-1));大小(100);[/asy]](https://latex.artofproblemsolving.com/1/1/a/11a69eb2f68ba08e0f212bacb515a331670c3d57.png)
![[asy] 导入 cse5; 大小(12cm); 笔 tpen = defaultpen + 1.337; 实际 a = 39/5.0; 实际 b = 39/7.0; 对 B = MP("B", (0,0), dir(200)); 对 A = MP("A", (9,0), dir(-80)); 对 C = MP("C", (12,0), dir(-20)); 对 K = (6,10.392); 对 M = (a*B+(12-a)*K) / 12; 对 N = (b*C+(12-b)*K) / 12; 绘制(B--M--N--C--cycle, tpen); 绘制(M--A--N--cycle); 填充(M--A--N--cycle, mediumgrey); 对 shift = (-20.13, 0);对 B1 = MP("B", B+shift, dir(200)); 对 A1 = MP("A", K+shift, dir(90)); 对 C1 = MP("C", C+shift, dir(-20)); draw(A1--B1--C1--cycle, tpen);[/asy]](https://latex.artofproblemsolving.com/8/6/1/8614a5c1f3561dd65964cf67fa56b23139e833c3.png)
![[asy] 对 A、B、C、D、E、F、R、S、T、X、Y、Z;dotfactor = 2;unitsize(.1cm);A = (0,0);B = (0,18);C = (0,36);//不要看这里D = (12*2.236, 36);E = (12*2.236, 18);F = (12*2.236, 0);draw(A--B--C--D--E--F--cycle);dot(" ",A,NW);dot(" ",B,NW);dot(" ",C,NW);dot(" ",D,NW);dot(" ",E,NW);dot(" ",F,NW);//不要看这里R = (12*2.236 +22,0); S = (12*2.236 + 22 - 13.4164,12); T = (12*2.236 + 22,24); X = (12*4.472+ 22,24); Y = (12*4.472+ 22 + 13.4164,12); Z = (12*4.472+ 22,0); draw(R--S--T--X--Y--Z--cycle); dot(" ",R,NW); dot(" ",S,NW); dot(" ",T,NW); dot(" ",X,NW); dot(" ",Y,NW); dot(" ",Z,NW); // sqrt180 = 13.4164 // sqrt5 = 2.236[/asy]](https://latex.artofproblemsolving.com/9/5/c/95cac62aaac35028476cf23e914b63412df36f70.png)

![[asy] 尺寸(200);默认笔(线宽(0.7));路径 laceL=(-20,-30)..张力 0.75 ..(-90,-135)..(-102,-147)..(-152,-150)..张力 2 ..(-155,-140)..(-135,-40)..(-50,-4)..张力 0.8 ..原点;路径 laceR=reflect((75,0),(75,-240))*laceL;绘制(原点--(0,-240)--(150,-240)--(150,0)--循环,灰色); for(int i=0;i<=3;i=i+1) { path circ1=circle((0,-80*i),5),circ2=circle((150,-80*i),5); unfill(circ1); draw(circ1); unfill(circ2); draw(circ2); } draw(laceL--(150,-80)--(0,-160)--(150,-240)--(0,-240)--(150,-160)--(0,-80)--(150,0)^^laceR,linewidth(1));[/asy]](https://latex.artofproblemsolving.com/a/6/1/a61b1059117868ce9afa4391360bfa9424211553.png)
![[asy] 对 A = (0,sqrt(850));对 B = (0,0);对 C = (sqrt(850),0);对 D = (sqrt(850),sqrt(850));绘制(A--B--C--D--循环);点因子 = 3;点(“$A$”,A,dir(135));点(“$B$”,B,dir(215));点(“$C$”,C,dir(305));点(“$D$”,D,dir(45));对 H = ((2sqrt(850)-sqrt(306))/6,sqrt(850));对 F = ((2sqrt(850)+sqrt(306)+7)/6,0);点(“$H$”,H,dir(90));点(“$F$”,F,dir(270));绘制(H--F);对 E = (0,(sqrt(850)-6)/2);对 G = (sqrt(850) ,(sqrt(850)+sqrt(100))/2); 点("$E$",E,dir(180)); 点("$G$",G,dir(0)); 绘制( E--G); 对 P = 扩展(H,F,E,G); 点(“$P$”,P,dir(60)); 标签(“$w$”, 交点(A--P , E--H )); 标签("$x$", 交点( B--P, E--F )); 标签("$y$", 交点( C--P, G--F ) ); 标签("$z$", 交点( D--P, G--H ));[/asy]](https://latex.artofproblemsolving.com/e/1/1/e1175984ad8a8e7468972e727cc95e27117b7d92.png)